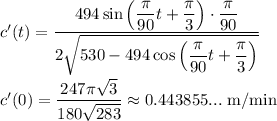Question

Two sides of a triangle have lengths 13 m and 19 m. The angle between them is increasing at a rate of 2°/min. How fast is the length of the third side increasing when the angle between the sides of fixed length is 60°? (Round your answer to three decimal places.)

Answer 1

9514 1404 393

Answer:

0.444 m/min

Explanation:

I find this kind of question to be answered easily by a graphing calculator.

The length of the third side can be found using the law of cosines. If the angle of interest is C, the two given sides 'a' and 'b', then the third side is ...

c = √(a² +b² -2ab·cos(C))

Since C is a function of time, its value in degrees can be written ...

C = 60° +2t° . . . . . where t is in minutes, and t=0 is the time of interest

Using a=13, and b=19, the length of the third side is ...

c(t) = √(13² +19² -2·13·19·cos(60° +2t°))

Most graphing calculators are able to compute a numerical value of the derivative of a function. Here, we use the Desmos calculator for that. (Angles are set to degrees.) It tells us the rate of change of side 'c' is ...

0.443855627418 m/min ≈ 0.444 m/min

_____

Additional comment

At that time, the length of the third side is about 16.823 m.

__

c(t) reduces to √(530 -494cos(π/90·t +π/3))

Then the derivative is ...

`c'(t)=\frac{494\sin{\left((\pi)/(90)t+(\pi)/(3)\right)}\cdot(\pi)/(90)}{2\sqrt{530-494\cos{\left((\pi)/(90)t+(\pi)/(3)}}\right)}}}\\\\c'(0)=(247\pi√(3))/(180√(283))\approx0.443855...\ \text{m/min}`

Answer 2

The third side is increasing at an approximate rate of about 0.444 meters per minute.

Explanation:

We are given a triangle with two sides having constant lengths of 13 m and 19 m. The angle between them is increasing at a rate of 2° per minute and we want to find the rate at which the third side of the triangle is increasing when the angle is 60°.

Let the angle between the two given sides be θ and let the third side be c.

Essentially, given dθ/dt = 2°/min and θ = 60°, we want to find dc/dt.

First, convert the degrees into radians:

`\displaystyle 2^\circ \cdot \frac{\pi \text{ rad}}{180^\circ} = (\pi)/(90)\text{ rad}`

Hence, dθ/dt = π/90.

From the Law of Cosines:

`\displaystyle c^2 = a^2 + b^2 - 2ab\cos \theta`

Since a = 13 and b = 19:

`\displaystyle c^2 = (13)^2 + (19)^2 - 2(13)(19)\cos \theta`

Simplify:

`\displaystyle c^2 = 530 - 494\cos \theta`

Take the derivative of both sides with respect to t:

`\displaystyle (d)/(dt)\left[c^2\right] = (d)/(dt)\left[ 530 - 494\cos \theta\right]`

Implicitly differentiate:

`\displaystyle 2c(dc)/(dt) = 494\sin\theta (d\theta)/(dt)`

We want to find dc/dt given that dθ/dt = π/90 and when θ = 60° or π/3. First, find c:

`\displaystyle \begin{aligned} c &= √(530 - 494\cos \theta)\\ \\ &=\sqrt{530 -494\cos (\pi)/(3) \\ \\ &= \sqrt{530 - 494\left((1)/(2)\right)} \\ \\&= \sqrt{283\end{aligned}`

Substitute:

`\displaystyle 2\left(√(283)\right) (dc)/(dt) = 494\sin\left((\pi)/(3)\right)\left((\pi)/(90)\right)`

Solve for dc/dt:

`\displaystyle (dc)/(dt) = (494\sin (\pi)/(3) \cdot (\pi)/(90))/(2√(283))`

Evaluate. Hence:

`\displaystyle \begin{aligned} (dc)/(dt) &= (494\left((√(3))/(2) \right)\cdot (\pi)/(90))/(2√(283))\\ \\ &= ((247√(3)\pi)/(90))/(2√(283))\\ \\ &= (247√(3)\pi)/(180√(283)) \\ \\ &\approx 0.444\text{ m/min}\end{aligned}`

The third side is increasing at an approximate rate of about 0.444 meters per minute.