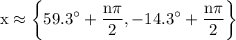Question

Find the general solution for:-


`sin\:x \:cos\: 3x+cos\:x\: sin\:3x=tan140`


~Please show your work
~Thank you!

Answer 1

`\rm \displaystyle x \approx \bigg \{ {59.3}^( \circ) + (n\pi)/(2) , - {14.3}^( \circ) + (n\pi)/(2) \bigg \}`

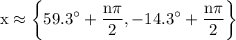
Explanation:

we would like to solve the following trigonometric equation:

`\rm \displaystyle \sin(x) \cos(3x) + \cos(x) \sin(3x) = \tan( {140}^( \circ) )`

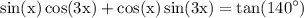
the left hand side can be rewritten using angle sum indentity of sin which is given by:

`\rm \displaystyle \sin( \alpha + \beta ) = \sin( \alpha ) \cos( \beta ) + \cos( \alpha ) \sin( \beta )`

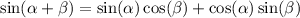
therefore Let

• 
  `\alpha = x`
• 
  `\beta = 3x`

Thus substitute:

`\rm \displaystyle \sin(x + 3x) = \tan( {140}^( \circ) )`

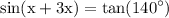
simplify addition:

`\rm \displaystyle \sin(4x) = \tan( {140}^( \circ) )`

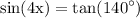
keep in mind that sin(t)=sin(π-t) saying that there're two equation to solve:

`\begin{cases} \rm \displaystyle \sin(4x) = \tan( {140}^( \circ) ) \\ \\ \displaystyle \sin(\pi - 4x) = \tan( {140}^( \circ) ) \end{cases}`

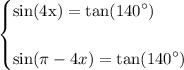
take inverse trig and that yields:

`\begin{cases} \rm \displaystyle 4x= { \sin}^( - 1) ( \tan( {140}^( \circ) ) ) \\ \\ \displaystyle \pi - 4x = { \sin}^( - 1)( \tan( {140}^( \circ) ) ) \end{cases}`

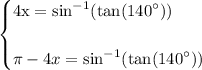
add π to both sides of the second equation and that yields:

`\begin{cases} \rm \displaystyle 4x= { \sin}^( - 1) ( \tan( {140}^( \circ) ) ) \\ \\ \displaystyle - 4x = { \sin}^( - 1)( \tan( {140}^( \circ) ) ) + \pi\end{cases}`

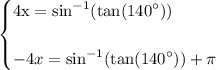
sin function has a period of 2nπ thus add the period:

`\begin{cases} \rm \displaystyle 4x= { \sin}^( - 1) ( \tan( {140}^( \circ) ) ) + 2n\pi\\ \\ \displaystyle - 4x = { \sin}^( - 1)( \tan( {140}^( \circ) ) ) + \pi + 2n\pi\end{cases}`

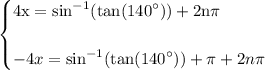
divide I equation by 4 and II by -4 which yields:

`\begin{cases} \rm \displaystyle x= \frac{ { \sin}^( - 1) ( \tan( {140}^( \circ) ) ) }{4} + (n\pi)/(2) \\ \\ \displaystyle x = - \frac{{ \sin}^( - 1)( \tan( {140}^( \circ) ) ) + \pi}{4} - (n\pi)/(2) \end{cases}`

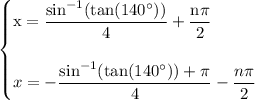
recall that,-½(nπ)=½(nπ) therefore,

`\begin{cases} \rm \displaystyle x= \frac{ { \sin}^( - 1) ( \tan( {140}^( \circ) ) ) }{4} + (n\pi)/(2) \\ \\ \displaystyle x = - \frac{{ \sin}^( - 1)( \tan( {140}^( \circ) ) ) + \pi}{4} + (n\pi)/(2) \end{cases}`

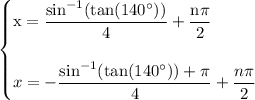
by using a calculator we acquire:

`\begin{cases} \rm \displaystyle x \approx - {14.3}^( \circ) + (n\pi)/(2) \\ \\ \displaystyle x \approx {59.3}^( \circ) + (n\pi)/(2) \end{cases}`

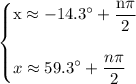
hence,

the general solution for: for the trig equation are

`\rm \displaystyle x \approx \bigg \{ {59.3}^( \circ) + (n\pi)/(2) , - {14.3}^( \circ) + (n\pi)/(2) \bigg \}`