Question

The equation y=2(x-1)^2-5y=2(x−1)

2
−5y, equals, 2, left parenthesis, x, minus, 1, right parenthesis, squared, minus, 5 is graphed in the xyxyx, y-plane. Which of the following statements about the graph is true?

Answer 1

Answer: It is symmetrical about x=1

Explanation:

Answer 2

(b) It is symmetrical about
`x = 1`

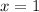
Explanation:

Given

`y = 2(x - 1)^2 - 5`

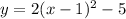
See attachment for options

Required

True statement about the graph

First, we check the line of symmetric

`y = 2(x - 1)^2 - 5`

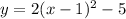
Expand

`y = 2(x^2 - 2x + 1) - 5`

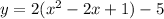
Open bracket

`y = 2x^2 - 4x + 2 - 5`

`y = 2x^2 - 4x -3`

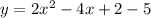
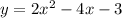
A quadratic equation
`y = ax^2 + bx + c` has the following line of symmetry

`x = -(b)/(2a)`

By comparison, the equation becomes:

`x = -(-4)/(2*2)`

`x = (4)/(4)`

`x = 1`

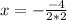
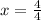
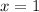
Hence, the line of symmetry is at:
`x = 1`

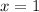
(b) is true.