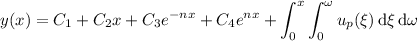Question

Find the form of the general solution of y^(4)(x) - n^2y''(x)=g(x)

Answer 1

The differential equation

`y^((4))-n^2y'' = g(x)`

has characteristic equation

r ⁴ - n ² r ² = r ² (r ² - n ²) = r ² (r - n) (r + n) = 0

with roots r = 0 (multiplicity 2), r = -1, and r = 1, so the characteristic solution is

`y_c=C_1+C_2x+C_3e^(-nx)+C_4e^(nx)`

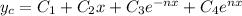
For the non-homogeneous equation, reduce the order by substituting u(x) = y''(x), so that u''(x) is the 4th derivative of y, and

`u''-n^2u = g(x)`

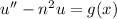
Solve for u by using the method of variation of parameters. Note that the characteristic equation now only admits the two exponential solutions found earlier; I denote them by u₁ and u₂. Now we look for a particular solution of the form

`u_p = u_1z_1 + u_2z_2`

where

`\displaystyle z_1(x) = -\int(u_2(x)g(x))/(W(u_1(x),u_2(x)))\,\mathrm dx`

`\displaystyle z_2(x) = \int(u_1(x)g(x))/(W(u_1(x),u_2(x)))\,\mathrm dx`

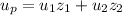
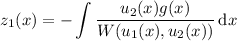
where W (u₁, u₂) is the Wronskian of u₁ and u₂. We have

`W(u_1(x),u_2(x)) = \begin{vmatrix}e^(-nx)&e^(nx)\\-ne^(-nx)&ne^(nx)\end{vmatrix} = 2n`

and so

`\displaystyle z_1(x) = -\frac1{2n}\int e^(nx)g(x)\,\mathrm dx`

`\displaystyle z_2(x) = \frac1{2n}\int e^(-nx)g(x)\,\mathrm dx`

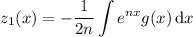
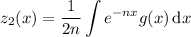
So we have

`\displaystyle u_p = -\frac1{2n}e^(-nx)\int_0^x e^(n\xi)g(\xi)\,\mathrm d\xi + \frac1{2n}e^(nx)\int_0^xe^(-n\xi)g(\xi)\,\mathrm d\xi`

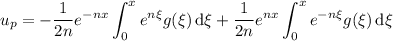
and hence

`u(x)=C_1e^(-nx)+C_2e^(nx)+u_p(x)`

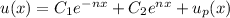
Finally, integrate both sides twice to solve for y :

`\displaystyle y(x)=C_1+C_2x+C_3e^(-nx)+C_4e^(nx)+\int_0^x\int_0^\omega u_p(\xi)\,\mathrm d\xi\,\mathrm d\omega`