Question

The regions bounded by the graphs of y=x2 and y=sin2x are shaded in the figure above. What is the sum of the areas of the shaded regions?

Answer 1

The sum of the area of the shaded regions = 0.248685

Step-by-step explanation:

The sum of the area of the shaded region is given as follows;

The point of intersection of the graphs are;

y = x/2

y = sin²x

∴ At the intersection, x/2 = sin²x

sinx = √(x/2)

Using Microsoft Excel, or Wolfram Alpha, we have that the possible solutions to the above equation are;

x = 0, x ≈ 0.55 or x ≈ 1.85

The area under the line y = x/2, between the points x = 0 and x ≈ 0.55, A₁, is given as follows

1/2 × (0.55)×0.55/2 ≈ 0.075625

The area under the line y = sin²x, between the points x = 0 and x ≈ 0.55, A₂, is given using as follows;

`\int\limits {sin^n(x)} \, dx = -(1)/(n) sin^(n-1)(x) \cdot cos(x) + (n-1)/(n) \int\limits {sin^(n-2)(x)} \, dx`

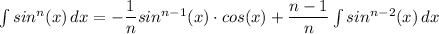
Therefore;

`A_2 = \int\limits^(0.55)_0 {sin^2x} \, dx = (1)/(2) \left [x -sin(x) \cdot cos(x) \right]_0 ^(0.55)`

∴ A₂ =1/2 × ((0.55 - sin(0.55)×cos(0.55)) - (0 - sin(0)×cos(0)) ≈ 0.0522

The shaded area,
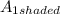`A_(1 shaded)` = A₁ - A₂ = 0.075625 - 0.0522 ≈ 0.023425

Similarly, we have, between points 0.55 and 1.85

A₃ = 1/2 × (1.85 - 0.55) × 1/2 × (1.85 - 0.55) + (1.85 - 0.55) × 0.55/2 = 0.78

For y = sin²x, we have;

`A_4 = \int\limits^(1.85)_(0.55) {sin^2x} \, dx = (1)/(2) \left [x -sin(x) \cdot cos(x) \right]_(0.55) ^(1.85) \approx 1.00526`

The shaded area,
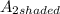`A_(2 shaded)` = A₄ - A₃ = 1.00526 - 0.78 ≈ 0.22526

The sum of the area of the shaded regions, ∑A =
`A_(1 shaded)` +
`A_(2 shaded)`

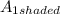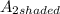
∴ A = 0.023425 + 0.22526 = 0.248685

The sum of the area of the shaded regions, ∑A = 0.248685