Question

Given that the triangle ABC is at A= (2,4) B= (5,9) C =(1,7) and if the triangle is reflected across the line y=1, what is the new position of point B?

Answer 1

We need not consider a whole triangle but just point B.

Before reflection we know that
`B(5,9)`.

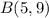Reflecting B over
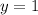`y=1` is relatively easy. First because its a reflection over the horizontal line the only coordinates that will change are y coordinates, while x coordinate will not change so half of the reflection is already done for us,

`B(5,a)`

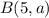
Now to what has changed, well currently the distance between 9 and 1 on the y axis is 8 up. But because we are reflecting the a must now be 8 down from 1 which means
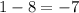`1 - 8 = -7` so our point is now
`\boxed{B(5,-7)}`.

Hope this helps :)

Answer 2

9514 1404 393

Answer:

(5, -7)

Explanation:

Reflection across the line y = c is accomplished by the transformation ...

(x, y) ⇒ (x, 2c -y)

For c=1 and point B, we have ...

B(5, 9) ⇒ B'(5, 2·1 -9) = B'(5, -7)

The image of point B is (5, -7).