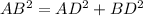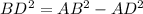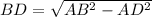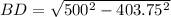Question

Does anybody know how to solve this?​

Answer 1

`AD = 403.75`

`CD = 115.70`

`BD = 294.93`

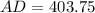
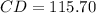
Explanation:

Given

The above triangle

Solving (a): AD

To solve AD, we consider triangle ACD.

Using cosine formula:

`cos(\angle A) = (AD)/(AC)`

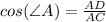
Where:

`\angle A =16`

`AC = 420`

So:

`cos(16) = (AD)/(420)`

`AD = 420 * cos16`

`AD = 420 * 0.9613`

`AD = 403.75`

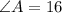
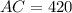
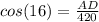
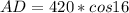
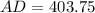
Solving (b): CD

Here, we make use of Pythagoras theorem:

`AC^2 = AD^2 + CD^2`

So:

`420^2 = 403.75^2 + CD^2`

`176400 = 163014.0625 + CD^2`

`CD^2 = 176400 - 163014.0625`

`CD^2 = 13385.9375`

`CD = \sqrt {13385.9375`

`CD = 115.70`

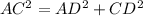
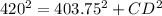
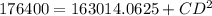
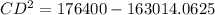
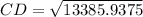
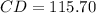
Solving (c): BD

Here, we make use of Pythagoras theorem:

`AB^2 = AD^2 + BD^2`

`BD^2 = AB^2 - AD^2`

`BD = \sqrt{AB^2 - AD^2`

`BD = \sqrt{500^2 - 403.75^2`

`BD = \sqrt{86985.9375`

`BD = 294.93`