Question

Find the indicated limit if it exists

Answer 1

`\displaystyle \lim_(x \to \infty) (x^2)/(1 + 3x + 5x^2) = (1)/(5)`

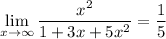
General Formulas and Concepts:

Calculus

• Limits

Explanation:

Step 1: Define

`\displaystyle \lim_(x \to \infty) (x^2)/(1 + 3x + 5x^2)`

Step 2: Determine Rule

If the highest power of x in a rational expression is the same both numerator and denominator, then the limit as x approached ∞ would be the highest term coefficient in the numerator divided by the highest term coefficient in the denominator.

Step 3: Identify

Numerator highest power: x²

• Coefficient: 1

Denominator highest power: 5x²

• Coefficient: 5

Step 4: Evaluate

Apply rule.

`\displaystyle \lim_(x \to \infty) (x^2)/(1 + 3x + 5x^2) = (1)/(5)`

Topic: AP Calculus AB/BC (Calculus I/II)

Unit: Limits

Book: College Calculus 10e