Question

When under a pressure of 9.5 atm, a piston has a volume of 1.7 liters. It the piston is compressed to a

volume of 1.1 liters, what will the new pressure be?
c. 8.90 atm
a. 5.08 atm
b. 6.15 atm
d. 14.7 atm

Answer 1

D

Step-by-step explanation:

according to boyle's law the temperature and pressure of a gas are inversely proportional given by the equation

p1v1=p2v2

In this case p1 is 9.5,v1 is 1.7,v2 is 1.1 and we have to find p2

9.5×1.7=p2×1.1

16.15/11=1.1p2/1.2

p2=14.7

I hope this helps

Answer 2

`\boxed {\boxed {\sf D.\ 14.7 \ atm}}`

Step-by-step explanation:

We are asked to find the pressure on a gas given a change in volume. We will use Boyle's Law which states the volume of a gas is inversely proportional to the pressure. The formula for this law is:

`P_1V_1=P_2V_2`

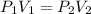
The gas originally has a pressure of 9.5 atmospheres and a volume of 1.7 liters.

`9.5 \ atm * 1.7 \ L = P_2V_2`

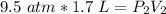
The gas is compressed to a volume of 1.1 liters, but the pressure is unknown.

`9.5 \ atm * 1.7 \ L = P_2* 1.1 \ L`

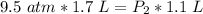
We want to find the new pressure, so we must isolate the variable P₂. It is being multiplied by 1.1 liters. The inverse operation of multiplication is division. Divide both sides of the equation by 1.1 L.

`\frac {9.5 \ atm * 1.7 \ L}{1.1 \ L} =( P_2* 1.1 \ L)/(1.1 \ L)`

`\frac {9.5 \ atm * 1.7 \ L}{1.1 \ L} =P_2`

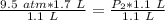
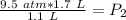
The units of liters cancel.

`\frac {9.5 \ atm * 1.7 }{1.1 } =P_2`

`\frac {16.15}{1.1} \ atm =P_2`

`14.6818181818 \ atm= P_2`

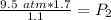
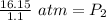
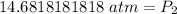
Round to the tenths place. The 8 in the hundredth place tells us to round the 6 up to a 7.

`14.7 \ atm \approx P_2`

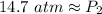
The new pressure at a volume of 1.1 liters is approximately 14.7 atmospheres.