Given:
A right prism has height 7½ and triangular bases with sides of length 5, 12, and 13.
To find:
The total surface area of the prism.
Solution:
We have,
Height of prism = 7½ = 7.5
Sides of triangular base are 5, 12, 13. These sides of Pythagorean triplets because
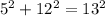
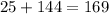
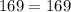
So, the base of the prism is a right triangle.
Area of a triangle is
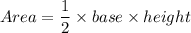
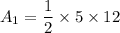
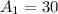
The area of the base is equal to the area of the top, i.e.,
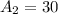 sq units.
sq units.
Perimeter of the base is
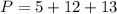
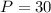
The curved surface area of the prism is

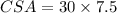
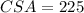
Now, the total area of the prism is
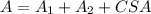
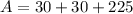
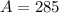
Therefore, the total surface area of the triangular prism is 285 square units.