Answer:
(5x+4)(5x-6)
Explanation:
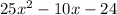
Separate into two parts, first realizing that the only two factor pairs for 25 are 5 and 5 or 1 and 25. Using 5 and 5 makes the most sense, so
(5x )(5x )
The other two numbers need to multiply to -24 and add up to -10.
Pairs that muliply to 24 could be 1 and 24, 2 and 12, 3 and 8, and 4 and 6. The more you factor the easier it will be to quickly discern which numbers to use. In this case, the right pair is 4 and 6. Because it needs to add up to -10, it should be 4 and -6 (because multiplying out, -30 + 20 = -10).
Thus, the final factored equation is (5x+4)(5x-6). You can check this by FOILing and making sure you get the original equation.