Question

Wich graph is the result of the reflection f(x) =1/4(8)^x across the y axis and then across the x- axis

Answer 1

I assume that the function is:

`f(x) = (1)/(4)*8^x`

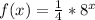
Now let's describe the general transformations that we need to use in this problem.

Reflection across the x-axis:

For a general function f(x), a reflection across the x-axis is written as:

g(x) = -f(x)

Reflection across the y-axis:

For a general function f(x), a reflection across the y-axis is written as:

g(x) = f(-x)

Then a reflection across the y-axis, and then a reflection across the x-axis is just:

g(x) = -(f(-x)) = -f(-x)

In this case, we have:

`f(x) = (1)/(4)*8^x`

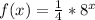
then:

`g(x) = -f(-x) = -(1)/(4)*8^(-x)`

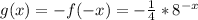
Now we can graph this, to get the graph you can see below: