Answer:
7 children and 3 adults
Explanation:
We should set up a system of equations for this problem, so let's say that:
x represents the number of children
y represents the number of adults
We know there is a total of 10 people, so:
x + y = 10
We also know that each adult had to pay $22.50 and each child had to pay $12.50. Their total amount paid is $155.00, so:
12.50x + 22.50y = 155.00
Simplifying this a bit:
12.5x + 22.5y = 155
Now, we can use the substitution method to solve this system of equations:
x + y = 10
y = 10 - x
12.5x + 22.5y = 155
12.5x + 22.5 (10 - x) = 155
12.5x + 225 - 22.5x = 155
12.5x - 22.5x = 155 - 225
-10x = 155 - 225
-10x = -70
x =
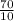
x = 7 children
Now we substitute back into the system of equations:
x + y = 10
7 + y = 10
y = 10 - 7
y = 3 adults