Question

Extra

A stone is dropped from a balloon that is descending at a uniform rate of
12 m/s when it is 1000 m from ground.
a. Calculate the velocity and position of the stone after 10 s and the time
it takes the stone to hit the ground.
b. Solve the same problem as for the case of a balloon rising at the given
velocity​

Answer 1

Assume that
`g =9.81\; \rm m\cdot s^(-1)`, and that the air resistance on the stone is negligible.

a.

Height of the stone:
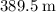`389.5\; \rm m` (above the ground.)

Velocity of the stone:
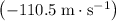`\left(-110.5\; \rm m \cdot s^(-1)\right)` (the stone is travelling downwards.)

b.

Height of the stone:
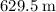`629.5\; \rm m` (above the ground.)

Velocity of the stone:
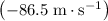`\left(-86.5\; \rm m \cdot s^(-1)\right)` (the stone is travelling downwards.)

Step-by-step explanation:

If air resistance on the stone is negligible, the stone would be accelerating downwards at a constant
`a = -g = -9.81\; \rm m \cdot s^(-2)`.

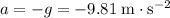Let
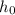`h_0` denote the initial height of the stone (height of the stone at
`t = 0`.)

Similarly, let
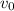`v_0` denote the initial velocity of the stone.

Before the stone reaches the ground, the height
`h` (in meters) of the stone at time
`t` (in seconds) would be:

`\displaystyle h(t) = -(1)/(2)\, g \cdot t^(2) + v_0 \cdot t + h_0`.

Similarly, before the stone reaches the ground, the velocity
`v` (in meters-per-second) of the stone at time
`t` (in seconds) would be:

`v(t) = -g\cdot t + v_0`.

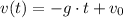In section a.,
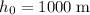`h_0 = 1000\; \rm m` while
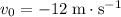`v_0 = -12\; \rm m\cdot s^(-1)` (the stone is initially travelling downwards.) Evaluate both
`h(t)` and
`v(t)` for
`t = 10\; \rm m \cdot s^(-1)`:

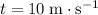`\begin{aligned} h(t) &= -(1)/(2)\, g \cdot t^(2) + v_0 \cdot t + h_0 \\ &= -(1)/(2)\ * 9.81\; \rm m\cdot s^(-2)* (10\; \rm s)^(2) \\&\quad\quad + \left(-12\; \rm m \cdot s^(-1)\right) * 10\; \rm s + 1000\; \rm m \\[0.5em] &= 389.5\; \rm m \end{aligned}`.

Indeed, the value of
`h(t)` at
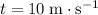`t = 10\; \rm m \cdot s^(-1)` is greater than zero. The stone hasn't yet hit the ground, and both the representation for the height of the stone and that for the velocity of the stone are valid.

`\begin{aligned} v(t) &= -g\cdot t + v_0 \\ &= -9.81\; \rm m\cdot s^(-2)* 10\; \rm s - 12\; \rm m\cdot s^(-1) \\ &= -110.5\; \rm m \cdot s^(-1) \end{aligned}`.

The value of
`v(t)` at
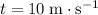`t = 10\; \rm m \cdot s^(-1)` is negative, meaning that the stone would be travelling downwards at that time.

In section b.,
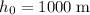`h_0 = 1000\; \rm m` while
`v_0 = 12\; \rm m\cdot s^(-1)` (the stone is initially travelling upwards.) Evaluate both
`h(t)` and
`v(t)` for
`t = 10\; \rm m \cdot s^(-1)`:

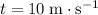`\begin{aligned} h(t) &= -(1)/(2)\, g \cdot t^(2) + v_0 \cdot t + h_0 \\ &= -(1)/(2)\ * 9.81\; \rm m\cdot s^(-2)* (10\; \rm s)^(2) \\&\quad\quad + 12\; \rm m \cdot s^(-1) * 10\; \rm s + 1000\; \rm m \\[0.5em] &= 629.5\; \rm m \end{aligned}`.

Verify that the value of
`h(t)` at
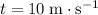`t = 10\; \rm m \cdot s^(-1)` is indeed greater than zero.

`\begin{aligned} v(t) &= -g\cdot t + v_0 \\ &= -9.81\; \rm m\cdot s^(-2)* 10\; \rm s + 12\; \rm m\cdot s^(-1) \\ &= -86.5\; \rm m \cdot s^(-1) \end{aligned}`.

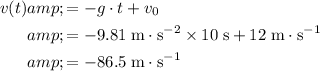Similarly, the value of
`v(t)` at
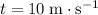`t = 10\; \rm m \cdot s^(-1)` is negative because the stone would be travelling downwards at that time.