Answer:
See below
Explanation:
We shall prove that for all
 . This tells us that 3 divides 4^n+5 with a remainder of zero.
. This tells us that 3 divides 4^n+5 with a remainder of zero.
If we let
 , then we have
, then we have
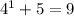 , and evidently,
, and evidently,
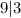 .
.
Assume that
 is divisible by
is divisible by
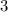 for
for
 . Then, by this assumption,
. Then, by this assumption,
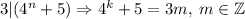 .
.
Now, let
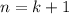 . Then:
. Then:

Since
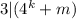 , we may conclude, by the axiom of induction, that the property holds for all
, we may conclude, by the axiom of induction, that the property holds for all
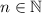 .
.