Question

Write the equation of a line in slope intercept form that passes through the two points. 4,-1 and 6,-7

Answer 1

A line has an equation of,

`y=mx+n`.

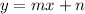m is called a slope

n is called y-intercept

We are also given two points
`(x_1,y_1)=(4,-1),(x_2,y_2)=(6,-7)`.

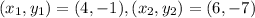We begin with computing the slope,

`m=(\Delta y)/(\Delta x)=(y_2-y_1)/(x_2-x_1)=(-7-(-1))/(6-4)=(-7+1)/(2)=(-6)/(2)=\boxed{-3}`

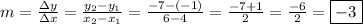
We have computed the slope m and our equation is almost done,

`y=-3x+n`

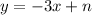
Next step is to find out what y-intercept n is. I will use point
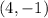`(4,-1)` and insert x and y it into already known equation, then solve for n,

`-1=-3(4)+n`

`-1=-12+n\implies n=\boxed{11}`

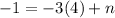
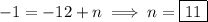
The reason I can insert coordinates of a point as x and y is because this particular point is in the line described by equation,

`\boxed{y=-3x+11}`

Hope this helps. :)