Answer:
1. The sides are: 38.5, 39.5, 40.5 and 41.5
2. Presently, Inzo is 15 years old and Miz is 10 years old
3.

4. Possible pairs are: (12, 14), (16, 18) and (20, 24)
5.
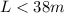
Explanation:
The question has lots of missing details. See the comment section for complete question
Solving (a):
Given
Shape: Quadrilateral
Sides: Consecutive
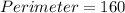
Let one of the sides be x. The other sides will be x + 1, x + 2 and x + 3.
So, the perimeter is:
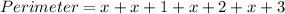
This gives:
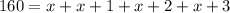
Collect Like Terms
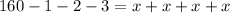

Divide both sides by 4
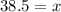
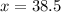
So, the sides are: 38.5, 39.5, 40.5 and 41.5
Solving (b):
Given
Presently:

Five years ago:
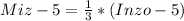 --- (Missing from the question)
--- (Missing from the question)
Required: Determine their present ages
Substitute
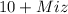 for Inzo in the second equation
for Inzo in the second equation

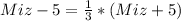
Multiply both sides by 3
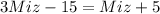
Collect Like Terms
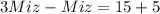
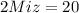
Divide both sides by 2
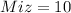
Substitute 10 for Miz in


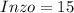
So presently, Inzo is 15 years old and Miz is 10 years old
Solving (3):
Given
Express Train:
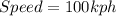
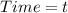
Local Train:
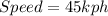

Required: Determine the distance between A and B
Distance is calculated as:
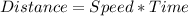
For the express train:
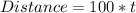
For the local train
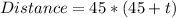
Distance between both stations are equal; So,
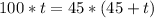
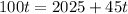
Collect Like Terms
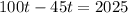
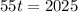
Divide by 55
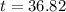
Substitute 36.82 for t in
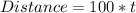
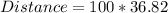

Solving (4):
Let the even numbers be: n and n + 2.
Sum greater than 24:
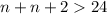
Sum less than 60:
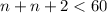
So, we have:
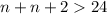
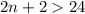
Collect Like Terms
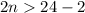
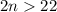

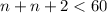
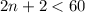
Collect Like Terms
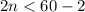
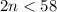
Divide by 2
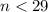
So, we have:
 and
and
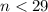
So, possible pairs are: (12, 14), (16, 18) and (20, 24)
Solving (5):
Given

Required: Find the possible lengths
Let the length be L.
Perimeter is calculated as:
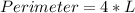
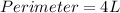
So, we have:

Divide through by 4
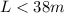
Hence, length is less than 38m (e.g. 37m)