Answer:
See Explanation
Explanation:
Your question is incomplete. I will take the complete question as:
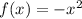
![Interval: [-1,1]](https://img.qammunity.org/2022/formulas/mathematics/high-school/zjn5cnvle0eigeaw1fgrm4np6fnogn1wii.png)
Required
Determine whether Rollie's theorem can be applied or not
First, find the coordinates of the endpoints on the closed interval [-1, 1]
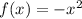
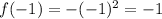 ------
------
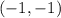
 ----
----
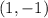
Find the derivative of f(x)
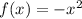
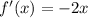
Calculate the slope (m) of the secant line i.e.
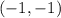 and
and
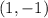
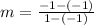
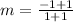
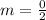
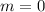
Set the derivative to the calculated slope:
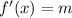
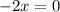

Plug in 0 for x in f'(0)
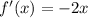

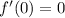
Since f'(0) is differentiable at x = 0. We can conclude that Rollie's theorem can be applied