Question

A line that includes the point (6,-4) has a slope of -1/2 what’s the equation in slope intercept form

Answer 1

The equation in the slope-intercept form will be:

• 
  `\:y=-(x)/(2)-1`

Explanation:

Given

• Point (6, -4)

• Slope m = -1/2

Important Tip:

The slope-intercept form of the line equation

`y = mx+b`

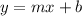
where

• 
  `m` represents the slope

• 
  `b` is the y-intercept

In our case,

• (x, y) = (6, -4)

• m = -1/2

Step 1 of 2

Determine the y-intercept b

substitute m = -1/2 and (x, y) = (6, -4) in the slope-intercept form of the line equation

`y = mx+b`

`-4\:=-\:(1)/(2)\left(6\right)+b`

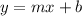
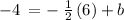
switch sides

`-(1)/(2)\left(6\right)+b=-4`

`-3+b=-4`

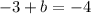
Add 3 to both sides

`-3+b+3=-4+3`

simplify

`b=-1`

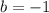
Thus, the y-intercept b = -1

Step 2 of 2

substitute the values

substitute b = -1 and m = 1/2 in the slope-intercept form of the line equation

`y = mx+b`

`y=-(1)/(2)x+\left(-1\right)`

`\:y=-(x)/(2)-1`

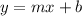
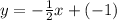
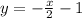
Conclusion:

Therefore, the equation in the slope-intercept form will be:

`\:y=-(x)/(2)-1`

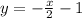
The graph of the line equation is also attached.