Question

Find the limit:
lim e−9x sin x
x→0

Answer 1

`\displaystyle \lim_(x \to 0) e^\big{-9x} \sin x = 0`

General Formulas and Concepts:

Pre-Calculus

• Unit Circle

Calculus

Limits

Limit Rule [Variable Direct Substitution]:
`\displaystyle \lim_(x \to c) x = c`

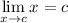
Explanation:

Step 1: Define

Identify

`\displaystyle \lim_(x \to 0) e^\big{-9x} \sin x`

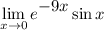
Step 2: Evaluate

1. Limit Rule [Variable Direct Substitution]:
   `\displaystyle \lim_(x \to 0) e^\big{-9x} \sin x = e^\big{-9(0)} \sin(0)`
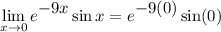
2. Simplify:
   `\displaystyle \lim_(x \to 0) e^\big{-9x} \sin x = 0`

Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Limits