Question

In a lunar experiment, a 950-g aluminum (920 J/(°Ckg)) sphere is dropped from the space probe while is 75 m above the Lunar ground. If the sphere’s temperature increased by 0.11°C when it hits the ground, what percentage of the initial mechanical energy was absorbed as thermal energy by the aluminum sphere?

Answer 1

13.759 % of the initial mechanical energy is lost as thermal energy.

Step-by-step explanation:

By the First Law of Thermodynamics we know that increase in internal energy of the object (
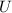`U`), in joules, is equal to the lost amount of the change in gravitational potential energy (
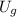`U_(g)`), in joules:

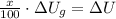`(x)/(100) \cdot \Delta U_(g) = \Delta U` (1)

Where
`x` is the percentage of the energy loss, no unit.

By definition of the gravitational potential energy and internal energy, we expand this equation:

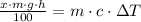`(x\cdot m \cdot g \cdot h)/(100) = m\cdot c\cdot \Delta T` (1b)

Where:

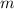`m` - Mass of the object, in kilograms.

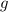`g` - Gravitational acceleration, in meters per square second.

`h` - Initial height of the object above the lunar ground, in meters.

`c` - Specific heat of aluminium, in joules per degree Celsius-kilogram.

`\Delta T` - Temperature increase due to collision, in degree Celsius.

If we know that
`m = 0.95\,kg`,
`g = 9.807\,(m)/(s^(2))`,
`h = 75\,m`,
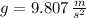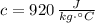`c = 920\,(J)/(kg\cdot ^(\circ)C)` and
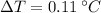`\Delta T = 0.11\,^(\circ)C`, then the percentage of energy loss due to collision is:

`x = (100\cdot c\cdot \Delta T)/(g\cdot h)`

`x = (100\cdot \left(920\,(J)/(kg\cdot ^(\circ)C) \right)\cdot (0.11\,^(\circ)C))/(\left(9.807\,(m)/(s^(2)) \right)\cdot (75\,m))`

`x = 13.759\,\%`

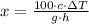
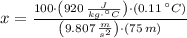
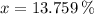
13.759 % of the initial mechanical energy is lost as thermal energy.