Question

Can any kind soul help me please​

Answer 1

`\displaystyle \theta = - {41.8}^( \circ) + 2n\pi, {210}^( \circ) + 2n\pi , {221.8}^( \circ) +2nπ , {330}^( \circ) +2nπ`

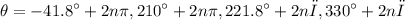
Explanation:

we would like to solve the following trigonometric equation:

`\displaystyle 6 { \sin}^(2) ( \theta) + 7 \sin( \theta) + 2 = 0`

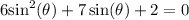
to do so we can let sinθ be u so it'll be easier to solve:

`\displaystyle 6 { u}^(2) + 7 u + 2 = 0`

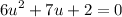
to notice is that it's a quadratic equation to solve the equation we can consider factoring method in this method we rewrite the middle term as sum or substraction of two different terms in that case 3u+4u can be mentioned:

`\displaystyle 6 { u}^(2) +3u + 4u + 2 = 0`

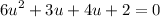
(continuing the method) well we can factor out the common terms and that yields:

`\displaystyle 3u(2 { u}^{} +1) + 2(2u + 1)= 0`

group:

`\displaystyle (3u+ 2)(2u + 1)= 0`

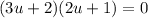
by Zero product property we acquire:

`\begin{cases}\displaystyle 3u+ 2 = 0 \\ 2u + 1= 0 \end{cases}`

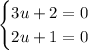
solve the equation for u:

`\begin{cases}\displaystyle u = - (2)/(3) \\ \\ u= - (1)/(2) \end{cases}`

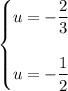
substitute back:

`\begin{cases}\displaystyle \sin( \theta) = - (2)/(3) \\ \\ \sin( \theta) = - (1)/(2) \end{cases}`

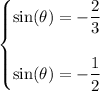
take inverse trig in both sides and that yields:

`\begin{cases}\displaystyle \theta = - {41.8}^( \circ), {221.8}^( \circ) \\ \\ \theta = {210}^( \circ) , {330}^( \circ) \end{cases}`

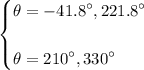
add period of 2nπ:

`\displaystyle \theta = - {41.8}^( \circ) + 2n\pi, {210}^( \circ) + 2n\pi , {221.8}^( \circ) +2nπ , {330}^( \circ) +2nπ`

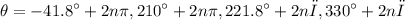
and we're done!