Answer:
Graph A → Equation A → Table B → Property D
Graph D → Equation B → Table A → Property B
Graph B → Equation C → Table C → Property E
Graph C → Equation D → Table E → Property A
Graph E → Equation E → Table D → Property C
Explanation:
Graph A corresponds to equation A, x·y = 2, and Table B → Property D
xy = 2 has a vertical asymptote at x = 0, and a horizontal asymptote at y = 0
y = 2/x, when x = 0, y = ∞
Graph D corresponds to equation B,
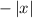 + 1 which corresponds to table A → Property B
+ 1 which corresponds to table A → Property B
The domain extends from (-∞, ∞), and [1, -∞)
Graph B corresponds to equation C, -(x + 2)² which gives the values in Table C and Property E wich is a horizontal shift of 2 units left and a reflection across the x-axis
Graph C corresponds to equation D, (1/3)·x - 2, which gives the values on Table E and Property A
The y-intercept of (1/3)·x - 2 = (0, -2), and the x-intercept = (6, 0)
Graph E corresponds to equation E, x³ = y, which gives the values on Table D Property C, y is x cubed