Answer:
About 3500 grams of tin.
Step-by-step explanation:
We want to determine amount of tin metal (in grams) that can be produced from smelting 4.5 kilograms of tin(IV) oxide.
First, write the chemical compound. Since our cation is tin(IV), it forms a 3+ charge. Oxygen has a 2- charge, so we will have two oxygen atoms. Hence, tin(IV) oxide is given by SnO₂.
By smelting it, we acquire elemental tin and oxygen gas. Hence:

(Note: oxygen is a diatomic element.)
The equation is balanced as well.
To convert from SnO₂ to only Sn, we can first convert from grams of SnO₂ to moles, use mole ratios to convert to moles of Sn, and then from there convert to grams.
Since Sn has a molar mass of 118.71 g/mol and oxygen has a molar mass of 15.999 g/mol, the molar mass of SnO₂ is:
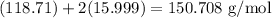
Therefore, given 4.5 kilograms of SnO₂, we can first convert this into grams using 1000 g / kg and then using the ratio:
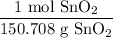
We can convert this into moles.
Next, from the chemical equation, we can see that one mole of SnO₂ produces exactly one mole of Sn (and also one mole of O₂). So, our mole ratio is:
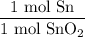
With SnO₂ in the denominator to simplify units.
Finally, we can convert from moles Sn to grams Sn using its molar mass:
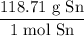
With the initial value and above ratios, we acquire:
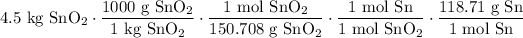
Cancel like units:
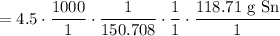
Multiply. Hence:
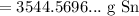
Since we should have two significant figures:
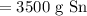
So, about 3500 grams of tin is produced from smelting 4.5 kg of tin(IV) oxide.