Question

Using a directrix of y = −2 and a focus of (2, 6), what quadratic function is created?

Answer 1

f(x) =
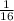`(1)/(16)` (x - 2)² + 2

Explanation:

From any point (x, y ) on the parabola the focus and the directrix are equidistant.

Using the distance formula

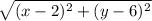`√((x-2)^2+(y-6)^2)` = | y + 2 | ← square both sides

(x - 2)² + (y - 6)² = (y + 2)² ← subtract (y + 2)² from both sides

(x - 2)² + (y - 6)² - (y + 2)² = 0 ← subtract (x - 2)² from both sides

(y - 6)² - (y + 2)² = - (x - 2)² ← expand left side using FOIL and simplify

y² - 12y + 36 - y² - 4y - 4 = - (x - 2)²

- 16y + 32 = - (x - 2)² ← subtract 32 from both sides

- 16y = - (x - 2)² - 32 ← divide all terms by - 16

y =
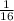`(1)/(16)` (x - 2)² + 2