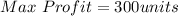Question

A product can be produced at a total cost (in dollars) of C (x )equals 0.05 x squared plus 10 x plus 1100, where x is the number of units produced. If the total revenue (in dollars) is given by R (x )equals 46 x minus 0.01 x squared, determine the number of units required to maximize the profit. Round your answer to 2 decimal places, if necessary. [A] units

Answer 1

`Max\ Profit=300units`

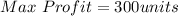
Explanation:

From the question we are told that:

`C(x)=0.05x^2+10x^2+1100`

`R(x)=4.6x-0.01x^2`

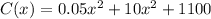
Generally the equation for Profit is is mathematically given by

`P(x)=R(x)-C(x)`

`P(x)=(4.6x-0.01x^2)-(0.05x^2+10x^2+1100`

`P(x)=36x-0.06x^2-1100`

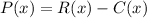
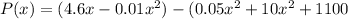
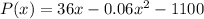
Differentiate P(x) we have

`P'(x)=36-2(0.06)x`

Equating to 0

`P'(x)=0`

`36-2(0.06)x=0`

`x=300units`

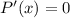
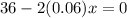
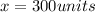
Second order differentiation, we have

`P''(x)=-0.12`

Therefore

This implies that

`P''(300)<0`

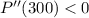
Hence, Maximum Profit is actualized at

`Max\ Profit=300units`