Question

A day trading firm closely monitors and evaluates the performance of its traders. For each $10,000 invested, the daily returns of traders at this company can be modeled by a Normal distribution with mean = $830 and standard deviation = $1,781.

(a) What is the probability of obtaining a negative daily return, on any given day? (Use 3 decimals.)
(b) Assuming the returns on successive days are independent of each other, what is the probability of having a negative daily return for two days in a row? (Use 3 decimals.)
(c) Give the boundaries of the interval containing the middle 80% of daily returns: (use 3 decimals) ( , )
(d) As part of its incentive program, any trader who obtains a daily return in the top 2% of historical returns receives a special bonus. What daily return is needed to get this bonus? (Use 3 decimals.)

Answer 1

a) 0.321 = 32.1% probability of obtaining a negative daily return, on any given day.

b) 0.103 = 10.3% probability of having a negative daily return for two days in a row.

c) (-$1449.68, $3109.68)

d) A bonus of $4,488.174 is needed.

Explanation:

Normal Probability Distribution

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
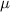`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

Normal distribution with mean = $830 and standard deviation = $1,781.

This means that
`\mu = 830, \sigma = 1781`

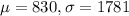
(a) What is the probability of obtaining a negative daily return, on any given day?

This is the p-value of Z when X = 0, so:

`Z = (X - \mu)/(\sigma)`

`Z = (0 - 830)/(1781)`

`Z = -0.466`

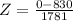
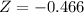
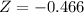`Z = -0.466` has a p-value 0.321.

0.321 = 32.1% probability of obtaining a negative daily return, on any given day.

(b) Assuming the returns on successive days are independent of each other, what is the probability of having a negative daily return for two days in a row?

Each day, 0.3206 probability, so:

`(0.321)^2 = 0.103`

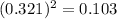
0.103 = 10.3% probability of having a negative daily return for two days in a row.

(c) Give the boundaries of the interval containing the middle 80% of daily returns

Between the 50 - (80/2) = 10th percentile and the 50 + (80/2) = 90th percentile.

10th percentile:

X when Z has a p-value of 0.1, so X when Z = -1.28.

`Z = (X - \mu)/(\sigma)`

`-1.28 = (X - 830)/(1781)`

`X - 830 = -1.28*1781`

`X = -1449.68`

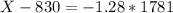
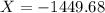
90th percentile:

X when Z has a p-value of 0.9, so X when Z = 1.28.

`Z = (X - \mu)/(\sigma)`

`1.28 = (X - 830)/(1781)`

`X - 830 = 1.28*1781`

`X = 3109.68`

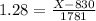
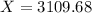
So

(-$1449.68, $3109.68)

d) As part of its incentive program, any trader who obtains a daily return in the top 2% of historical returns receives a special bonus. What daily return is needed to get this bonus?

The 100 - 2 = 98th percentile, which is X when Z has a p-value of 0.98, so X when Z = 2.054.

`Z = (X - \mu)/(\sigma)`

`2.054 = (X - 830)/(1781)`

`X - 830 = 2.054*1781`

`X = 4488.174`

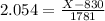
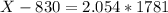
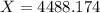
A bonus of $4,488.174 is needed.