Answer:
The output when x = -9 is f(x) = 187.
Explanation:
We are given a function and asked to find the output of that function.
- The input of a function refers to a value that is substituted into the function in order to simplify it to a final value.
- The output of a function is the value that is achieved when the input is substituted into the equation and the function is evaluated.
Our standard function is in the form of a quadratic equation.
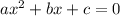
Let's check for a change in the presentation of the first value in the equation.
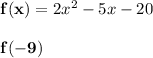
We see that x becomes -9. We also know that from conventional algebra, we need to make this change throughout the entire equation. Therefore, since we changed the x in f(x), we need to change it in 2x² - 5x - 20 as well.

Now, it's time to simplify this function. Let's first simplify the first term of the function:
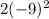 .
.
Let's follow PEMDAS in order to simplify the term.
P - Parentheses
E - Exponents
M - Multiplication
D - Division
A - Addition
S - Subtraction
When using this acronym, make sure that all operations are performed left to right.
We see that -9 is raised to the power of 2, so we square -9. Otherwise, we carry out the following operation.
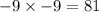
Then, we see that 2 is multiplied into this value. Therefore, we multiply 81 by 2.
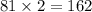
Now, we need to subtract the product of 5 and -9.
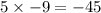
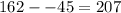
Finally, we subtract 20 from this value.
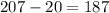
Therefore, the value of f(-9) is 187.