Question

Two men pull on a 31-kg box with forces 8.3 N and 6.6 N in opposite directions.

Find the resultant acceleration of the box and the direction in which the box moves.

Please HURRY

Answer 1

0.054m/s²

Step-by-step explanation:

Here we are given that a 31kg block is being pulled by two men who apply forces of 8.3N and 6.6N in opposite directions .

So we know that ,

`\sf \longrightarrow \boxed{\red{\rm F_(net)= √(F_1^2+F_2^2+2F_1F_2cos\theta )}}`

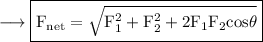
where
`\theta` is the angle between the forces .

• Since the forces act in opposite directions , the angle between them will be 180° .

`\sf \longrightarrow F_(net)= √( (8.3N)^2+(6.6N)^2+2(8.3)(6.6)(cos180^o))\\`

• Value of cos 180° = -1

`\sf \longrightarrow F_(net)= √( (8.3N)^2+(6.6N)^2+2(8.3)(6.6)(-1))\\`

`\sf \longrightarrow F_(net)= √( (8.3N)^2+(6.6N)^2-2(8.3)(6.6))\\`

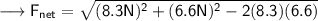
• RHS is in form of (a-b)² = a² + b² -2ab inside the square root .

`\sf \longrightarrow F_(net)=√(( 8.3N - 6.6N)^2) \\`

`\sf \longrightarrow F_(net)= 8.3N - 6.6N \\`

`\sf \longrightarrow \red{ F_(net)= 1.7N} \\`

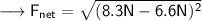
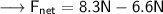
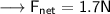
Now we know that ,

`\sf \longrightarrow F_(net)= mass * acceleration \\`

`\sf \longrightarrow 1.7N = 31kg * a\\`

`\sf \longrightarrow a =(1.7N)/(31kg)\\`

`\sf \longrightarrow \underline{\boxed{\bf accl^n = 0.054 m/s^2}} \\`

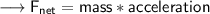
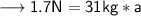
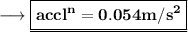
• And the box will move in the direction of application of 8.3N force .