Question

If m ∆ n means m²-2mm+n³, determine the value of 3∆2.​

Answer 1

`\large\underline{\underline{ \sf\maltese Correct \ Question :- }}`

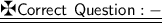
If m ∆ n means m²-2mm+n², determine the value of 3∆2.

`\large\underline{\underline{ \sf\maltese Answer :- }}`

Here we are given that m ∆ n means m² -2mn +n². And we need to find the value of 3∆2 . So let's find out what does the " ∆ " operator does.

Here we can see that m² - 2mn - n² is the whole square of ( m - n)² . A inference can be taken out that the operator " ∆ " first subtracts the two numbers and then squares the obtained value to get the result . That is ,

`\sf\implies m \Delta n = ( m - n )^2`

Now similarly let's simplify out 3 ∆ 2.

Step 1 : Subtract the two numbers :-

This means we need to subtract 2 from 3 . This will give result as ,

`\sf\implies Result_1 = 3 - 2 =\pink{1}`

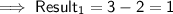
Step 2 : Square the obtained value :-

Here , the value obtained from step 1 is 1 and the square of 1 is 1 . So our final result would be,

`\sf\implies Result_((final))= 1^2 = \boxed{\pink{ 1 }}`

Answer 2

since m▲n=m^2-2mn+n^3

3▲2=3^2-2*3*2+2^3

=9-12+8

=17-12

=15

Done!