Question

a gym class has 10 boys and 12 girls. how many ways can a team of 6 be selected if the team must have the same number of boys and girls

Answer 1

The number of ways of selecting the team is 26,400 ways.

Explanation:

Given;

total number boys in the gym, b = 10 boys

total number of girls in the gym, g = 12 girls

number of team to be selected, n = 6

If there must equal number of boys and girls in the team, then the team must consist of 3 boys and 3 girls.

Number of ways of choosing 3 boys from the total of 10 =
`10_C_3`

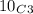
Number of ways of choosing 3 girls from a total of 12 =
`12_C_3`

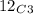
The number of ways of combining the two possibilities;

`n = 10_C_3 * 12_C_3\\\\n = (10!)/(7!3!) \ * \ (12!)/(9!3!) \\\\n = (10* 9 * 8)/(3* 2) \ * \ (12* 11 * 10)/(3* 2) \\\\n = 120 * 220\\\\n = 26,400 \ ways`

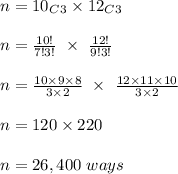
Therefore, the number of ways of selecting the team is 26,400 ways.