Question

Recently, mumps outbreaks have become more common, with many occurring among individuals 18-24 years of age living on college campuses. Two doses of the measles-mumps-rubella (MMR) vaccine are recommended for protection from mumps. Herd immunity refers to the proportion of individuals that must be immune to effectively prevent the spread of disease through a population. In order to prevent the spread of mumps, at least 96% of people in a community must have received two doses of the MMR vaccine.

a) Suppose that 94% of undergraduate students in the United States report having received two doses of the MMR vaccine. What is the probability that in one upperclassman House at MIT, enough students are vaccinated to achieve herd immunity? There are approximately 400 students in any house.

b) Calculate the probability that herd immunity is achieved in all 12 Houses.

c) Discuss the validity of the assumptions required to make the calculation in part i.

Answer 1

a) 0.0465 = 4.65% probability that in one upperclassman House at MIT, enough students are vaccinated to achieve herd immunity.

b)
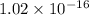`1.02 * 10^(-16)` probability that herd immunity is achieved in all 12 Houses.

c) We needed that
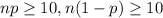`np \geq 10, n(1-p) \geq 10`, which was achieved.

Explanation:

To solve this question, we need to understand the binomial distribution, normal probability distribution and the central limit theorem.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

And p is the probability of X happening.

Normal Probability Distribution:

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

Central Limit Theorem

For a proportion p in a sample of size n, as long as
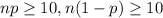`np \geq 10, n(1-p) \geq 10` the sampling distribution of the sample proportion will be approximately normal with mean
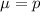`\mu = p` and standard deviation
`s = \sqrt{(p(1-p))/(n)}`

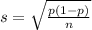
a) Suppose that 94% of undergraduate students in the United States report having received two doses of the MMR vaccine. What is the probability that in one upperclassman House at MIT, enough students are vaccinated to achieve herd immunity? There are approximately 400 students in any house.

We have that
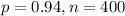`p = 0.94, n = 400`. So

`np = 400*0.94 = 376 \geq 10`

`n(1-p) = 400*0.06 = 24 \geq 10`

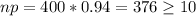
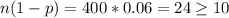
The standard deviation is:

`s = \sqrt{(0.94*0.06)/(400)} = 0.0119`

The probability is 1 subtracted by the pvalue of Z when X = 0.96. So

`Z = (X - \mu)/(\sigma)`

By the Central Limit Theorem

`Z = (X - \mu)/(s)`

`Z = (0.96 - 0.94)/(0.0119)`

`Z = 1.68`

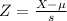
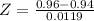
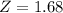
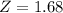`Z = 1.68` has a pvalue of 0.9535

1 - 0.9535 = 0.0465 = 4.65% probability that in one upperclassman House at MIT, enough students are vaccinated to achieve herd immunity.

b) Calculate the probability that herd immunity is achieved in all 12 Houses.

Now, we use the binomial distribution. This is
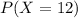`P(X = 12)` when
`n = 12` with
`p = 0.0465`. So

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 12) = C_(12,12).(0.0465)^(12).(0.9535)^(0) = 1.02 * 10^(-16)`

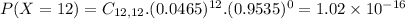
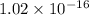`1.02 * 10^(-16)` probability that herd immunity is achieved in all 12 Houses.

c) Discuss the validity of the assumptions required to make the calculation in part i.

We needed that
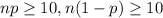`np \geq 10, n(1-p) \geq 10`, which was achieved.