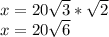Question

Use the figure to find x.

Answer 1

`x = 20\sqrt6`

Explanation:

The triangle with the side that has a measure of (
`10 √(3)`) is a (30 - 60 - 90) triangle. This means that its angles are (30), (60), and (90) degrees. One property of a (30 - 60 -90) triangle is the ratio of its sides. This ratio, in simple terms, can be defined as the following:

angle : opposite side

`30 : z\\60 : z√(3)\\90 : 2z`

Use this property here to find the measure of the side opposite the (90) degree angle, that is shared between the two triangles.

This side is opposite the (30) degree angle, therefore, multiply this side by (2) will yield the measure of the side opposite the (90) degree angle. Therefore the side opposite the (90) degree angle has the following measure:

`20√(3)`

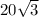
The triangle with a side of (x) is a (45 - 45 - 90) triangle. This means that its angles have a measure of (45 - 45 - 90). The ratios of the sides of a (45 - 45 - 90) triangle are as follows:

angle : opposite side

`45:y\\45:y\\90:y√(2)`

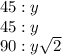
Apply this ratio here; multiply the side shared between the (30 - 60 - 90) triangle and (45 - 45- 90) triangle by (
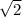`√(2)`) in order to get the side with a measure of (x). When this is done, one gets the following result:

`x = 20√(3)*√(2)\\x = 20√(6)`

Answer 2

`20√(6)`

Explanation:

In all 30-60-90 triangles, the side lengths are in the ratio
`x:x√(3):2x`, where
`2x` is the hypotenuse and
`x` is the side opposite to the 30 degree angle. Therefore, the hypotenuse of the 30-60-90 triangle (left) is
`2\cdot 10√(3)=20√(3)`. This hypotenuse also represents one leg of the 45-45-90 triangle.

In all 45-45-90 triangles, the side lengths are in ratio
`x:x:x√(2)` where
`x√(2)` is the hypotenuse of the triangle. Therefore, since
`x` is the hypotenuse of the triangle marked and
`20√(3)` is one of the legs, the value of
`x` must be:

`20√(3)\cdot √(2)=\boxed{20√(6)}`