Question

Rate of change or rate of change

A farmer has 80 feet of wire mesh to surround a rectangular pen.
A) Express the area A of the pen as a function of x, also draw the figure of A indicating the admissible values ​​of x for this problem.
B) What are the dimensions of the maximum area pen?

Answer 1

Explanation:

A). Let the dimensions of the rectangular pen are,

Length = l

Width = x

Since, farmer has the wire measuring 80 feet to surround the the pen.

Perimeter of the pen = 80 feet

2(l + x) = 80

l + x = 40

l = 40 - x ------(1)

Area of the rectangular pen = Length × width

= lx

By substituting the value of l from equation (1),

Area (A) of the pen will be modeled by the expression,

A = (40 - x)

A = 40x - x²

B). For maximum area of the pen,

Derivative of the area = 0

`(d)/(dx)(A)=0`

`(d)/(dx)(A)=(d)/(dx)(40x-x^2)`

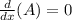
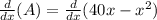
= 40 - 2x

And (40 - 2x) = 0

x = 20

Therefore, width of the pen = 20 feet

And length of the pen = 40 - 20

= 20 feet

Dimensions of the pen should be 20 feet by 20 feet.