Answer:
1.

2.
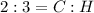
3.
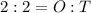
4.
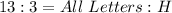
5.

Explanation:
See comment for complete question.
We have the following letters and their frequencies
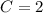
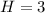
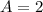
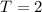

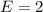
Solving (1): 1.
![3 : 2 = H : [\ ]](https://img.qammunity.org/2022/formulas/mathematics/high-school/x0fj9rl9xiuou7w009vvmxonkmtmp0uzv7.png)
From the alphabets listed above:
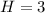 ,
,
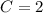
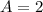
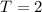

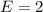
So, the 3:2 can be H : any of the alphabets. For this solution, we use:

Solving (2)
![2 : 3 = [\ ] : H](https://img.qammunity.org/2022/formulas/mathematics/high-school/g009bkw4xsb05lsrjnzcyda0qffsy3j0xg.png)
This is the inverse of (1) solved above.
So 2 : 3 is:
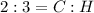
Solving (3)
![2 : 2 = O : [\ ]](https://img.qammunity.org/2022/formulas/mathematics/high-school/yybno86zb11l2tpef7jvxbemtbwx4285kb.png)
From the alphabets listed above:
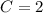
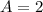
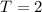

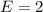
Any selected alphabet will represent 2 : 2
So, we have:
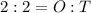 or O : any of C, A and E
or O : any of C, A and E
Solving (4):.
![13 : 3 = [\ ] : H](https://img.qammunity.org/2022/formulas/mathematics/high-school/scy7ii8rt9l1jgt9wl5im1loc0n87kxb2m.png)
The total of all alphabet in the given word is: 13.
So,
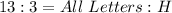
Solving (5):
![2 : 13 = E : [\ ]](https://img.qammunity.org/2022/formulas/mathematics/high-school/eawxinvjqr34d2fd8urvdmwd179rfxokbs.png)
The total of all alphabet in the given word is: 13.
So,
