Question

The length of a rectangle is 12x + 7 and the width is 6x – 5. Jason cut a square with side length of (x + 2) from the rectangle. Which expression represents the remaining area of the original rectangle?

Answer 1

The remaining area of the original rectangle can be expressed as: length = 11x + 5 & width = 5x - 7

Explanation:

Jason cut a square out of a rectangle which means the new rectangle will be smaller than the original one. To find the new length and width of the rectangle, we subtract (x + 2) from the original length and width respectively. So we'll have:

`new \: length = 12x \: + 7 \: - (x + 2) \\ = 12x \: + 7 - x - 2`

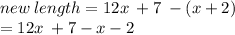
When you expand and open the bracket, (x + 2) will become - x - 2. Now, collect like terms:

`= 12x - x + 7 - 2 \\ = 11x \: + 5.`

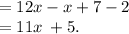
The new length will be = 11x + 5

To find the new width of the rectangle, we'll have:

`new \: width \: = 6x - 5 - (x + 2) \\ = 6x - 5 - x - 2 \\ = 6x - x - 5 - 2 \\ = 5x - 7.`

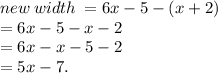
So we have (5x - 7) as the new width.