Answer:
0.1108 = 11.08% probability that there are exactly 3 faulty candy bars among the seven.
Explanation:
The bars are chosen without replacement, which means that the hypergeometric distribution is used to solve this question.
Hypergeometric distribution:
The probability of x successes is given by the following formula:
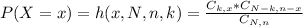
In which:
x is the number of successes.
N is the size of the population.
n is the size of the sample.
k is the total number of desired outcomes.
Combinations formula:
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.
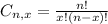
In this question:
60 total candies means that
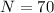
12 are faulty, which means that
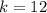
Seven are chosen, so

What is the probability that there are exactly 3 faulty candy bars among the seven?
This is
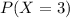 . So
. So
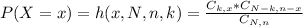
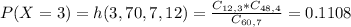
0.1108 = 11.08% probability that there are exactly 3 faulty candy bars among the seven.