Answer: Either 4/3 or -4/3
============================================================
Step-by-step explanation:
Let r be the common ratio. Also, we'll make r nonzero, i.e.
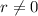
Multiplying this common ratio by any term gets us the next term of the geometric sequence.
16/9 is the first term, so that makes (16/9)*r the second term
Since (16/9)r is the second term, the third term is (16/9)r*r = (16/9)r^2
Set this equal to 1 and solve for r.
(16/9)r^2 = 1
r^2 = 1*(9/16)
r^2 = 9/16
r = sqrt(9/16) or r = -sqrt(9/16)
r = 3/4 or r = -3/4
Now that we know what r is, we can determine the second term
If r = 3/4, then,
(16/9)*r = (16/9)*(3/4) = 4/3
Or if r = -3/4, then,
(16/9)*r = (16/9)*(-3/4) = -4/3
So the second term is either 4/3 or -4/3 depending on which r value you go for.