Question

A compound contains 7.79% C and 92.21% Cl. What is the molecular formula if it has a molar mass of 152g?​

Answer 1

Molecular Formula: CCl4

Step-by-step explanation:

First, in order to find the molecular formula, we have to find the empirical formula.

We need to convert the percentages of each element to grams, and then moles. Usually, for problems like these, since we don't already have the chemical formula, we assume that the percents are in grams...

7.79% C becomes 7.79 grams of Carbon, and

92.21% Cl becomes 92.21 grams of Chlorine.

Now that we have grams, we can convert to moles.

The amu of Carbon is 12.01 g, and the amu of Chlorine is 35.45 g.

Carbon in moles =
`(7.79 g C)((1 mol C)/(12.01 g C)) =0.6486261449 = 0.65 \\`

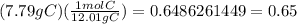
Chlorine in moles =
`(92.21 g Cl)((1 mol Cl)/(35.45 g Cl)) = 2.60112835 = 2.6`

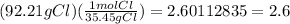
Now that we have the moles, we want to keep these as subscripts and write them into an empirical formula.

Since these numbers are in decimal formula, we have to divide them by the smallest mole in order to get a whole-number for our subscripts.

0.65/0.65 = 1, so the subscript for carbon is 1 (which can just be written as C with nothing next to it)

2.6/0.65 = 4, so the subscript for chlorine is 4 (which can be written as Cl4)

Now we will write our empirical formula as
`C Cl_(4)`.

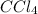In order to find our molecular formula, we will take the molecular mass (aka the molecular formula's molar mass which is 152) and divide by the mass from the empirical formula. Then we will get another whole-number multiple that we will multiply our subscripts by in order the numbers for the subscripts for our molecular formula.

The total mass from the empirical formula is 153.81

[12.01 + 4(35.45) = 153.81]

152/153.81 = 0.9882322346 which can be rounded to 0.99 or just 1.0..

When we multiply our subscripts by 1.0, they equal 1 and 4 (the same numbers as before), which means that our empirical formula and molecular formulas are the same.

Empirical Formula: CCl4

Molecular Formula: CCl4

Answer 2

For convenience, assume that we have 100 g of this unknown compound. Given the percent composition, we can then assume that we have 7.79 g C and 92.21 g Cl.

Next, convert these masses to moles.

For C: (7.79 g) ÷ (12.01 g/mol) = 0.6486 mol C.

For Cl: (92.21 g) ÷ (35.453 g/mol) 2.601 mol Cl.

Now, we divide each molar quantity by the smaller of the two (in this case, that'd be 0.6486 mol C) to obtain a whole-number subscript—what we're doing here is finding the ratio of the moles of each element.

0.6486 mol C/0.6486 = 1

2.601 mol Cl/0.6486 = 4.010 ≈ 4.

What we have now are the mole ratios that would comprise the subscripts of the empirical formula of our compound, which would be CCl₄. But the empirical formula and molecular formula are not necessarily the same; the empirical formula gives us the simplest ratios between the atoms, but the molecular formula (and the actual compound) may have a ratio that's larger. To figure out the factor by which our ratios differ, we divide the molar mass of the actual compound by the molar mass of the empirical compound. The molar mass of the actual compound is given to us as 152 g/mol (that's a typo in the question); the molar mass of the empirical compound is 153.82 g/mol.

Now, just from looking at it, we should be able to tell that the quotient is approximately equal to one. Precisely, it's 152/153.82 = 0.988 ≈ 1.

So, in this case, our empirical and molecular formulae are the same. Thus, the molecular formula of our compound is CCl₄.