Question

An advertising agency notices that approximately 1 in 50 potential buyers of a product ssees a given magazine ad, and 1 in 5 sees a corresponding ad on television. One in 100 see both. One in 3 actually purchases the product after seeing the ad, 1 in 10 without seeing it. What is the probability that a randomly selected potential customer will purchase the product

Answer 1

0.1490 = 14.90% probability that a randomly selected potential customer will purchase the product.

Explanation:

Probability of seeing the ad:

I am going to find this probability using Venn Sets.

I am going to say that:

Event A: Sees a magazine ad.

Event B: Sees a television ad.

Approximately 1 in 50 potential buyers of a product sees a given magazine ad

This means that
`P(A) = (1)/(50) = 0.02`

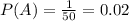
1 in 5 sees a corresponding ad on television.

This means that
`P(B) = (1)/(5) = 0.2`

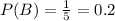
One in 100 see both.

This means that
`P(A \cap B) = (1)/(100) = 0.01`

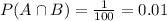
The probability of seeing the ad is the probability of seeing at least one of those(magazine or tv), which is:

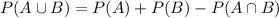`P(A \cup B) = P(A) + P(B) - P(A \cap B)`. So

`P(A \cup B) = 0.2 + 0.02 - 0.01`

`P(A \cup B) = 0.21`

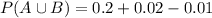
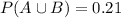
Probability of purchasing the product.

0.21 = 21% of customers see the add. Of those, 1/3 = 0.3333 buy the product.

1 - 0.21 = 0.79 = 79% of customers dont see the add. Of those, 1/10 = 0.1 buy the product. So

`p= 0.21*0.3333 + 0.79*0.1 = 0.1490`

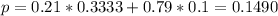
0.1490 = 14.90% probability that a randomly selected potential customer will purchase the product.