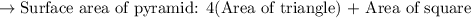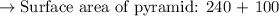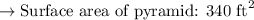Answer:
340 ft²
Explanation:
The surface area of a shape is basically the area of its faces.
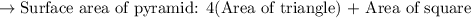
1. First, Let's find the area of the triangles. To find the area of a triangle, we need to multiply the height and the base and then divide the product by 2.
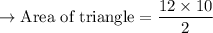
Now, let's find the area of the triangle by simplifying the RHS.
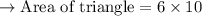
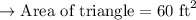
Since there are four triangles, we need to further multiply the area of the triangle by 4 to find the area of four triangles.
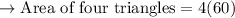
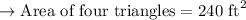
2. Now, let's find the area of the square. To find the area of the square, we need to square the side length.
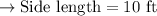

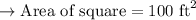
3. Lastly, let's find the surface area. To find the surface area of the figure, we need to sum up the area of the triangles and the area of the square.