Question

The mass, m grams, of a radioactive substance, present at time t days after first being observed, is given by the formula m=24e^-0.02t. Find

(i) the value of m when t=30.
(ii) the value of t when the mass is half of its value at t=0.
(iii) the rate at which the mass is decreasing when t=50.

Answer 1

(i) The value of m when t = 30 is 13.2

(ii) The value of t when the mass is half of its value at t=0 is 34.7

(iii) The rate of the mass when t=50 is -0.18

Explanation:

(i) The m value when t = 30 is:

`m = 24e^(-0.02t) = 24e^(-0.02*30) = 13.2`

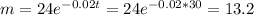
Then, the value of m when t = 30 is 13.2

(ii) The value of the mass when t=0 is:

`m_(0) = 24e^(-0.02t) = 24e^(-0.02*0) = 24`

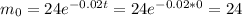
Now, the value of t is:

`ln((m_(0)/2)/(24)) = -0.02t`

`t = -(ln((24)/(2*24)))/(0.02) = 34.7`

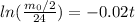
Hence, the value of t when the mass is half of its value at t=0 is 34.7

(iii) Finally, the rate at which the mass is decreasing when t=50 is:

`(dm)/(dt) = (d)/(dt)(24e^(-0.02t)) = 24(e^(-0.02t))*(-0.02) = -0.48*                            (e^(-0.02*50)) = -0.18`

Therefore, the rate of the mass when t=50 is -0.18.

I hope it helps you!