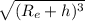Question

A satellite of mass m, originally on the surface of the Earth, is placed into Earth orbit at an altitude h. (a) Assuming a circular orbit, how long does the satellite take to complete one orbit

Answer 1

T = 5.45 10⁻¹⁰
`√((R_e + h)^3)`

Step-by-step explanation:

Let's use Newton's second law

F = ma

force is the universal force of attraction and acceleration is centripetal

G m M / r² = m v² / r

G M / r = v²

as the orbit is circular, the speed of the satellite is constant, so we can use the kinematic relations of uniform motion

v = d / T

the length of a circle is

d = 2π r

we substitute

G M / r = 4π² r² / T²

T² =
`(4\pi ^2 )/(GM) \ r^3`

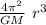
the distance r is measured from the center of the Earth (Re), therefore

r = Re + h

where h is the height from the planet's surface

let's calculate

T² =
`(4\pi ^2)/( 6.67 \ 10^(-11) \ 1.991 \ 10^(30))` (Re + h) ³

T =
`\sqrt{29.72779 \ 10^(-20)} \ \sqrt[2]{R_e+h)^3}`

T = 5.45 10⁻¹⁰
`√((R_e + h)^3)`