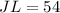Answer:
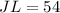
Explanation:
We are given that K is the midpoint of JL. Using this information, we want to find JL.
By the definition of midpoint, this means that:
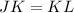
Substitute them for their equations:
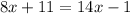
Solve for x. Subtract 8x from both sides:
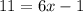
Add 1 to both sides:
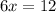
And divide both sides by 6. Hence:
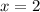
JL is the sum of JK and KL. Hence:

Since JK = KL, substitute either one for the other:
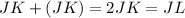
Substitute JK for its equation:

Since we know that x = 2:
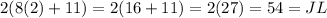
Thus: