Question

Mike and Andy are each reading the same book. Mike read 2/4 of the book on Tuesday and 1/3 of the book on Wednesday. Andy read 1/2 of the book on Tuesday and 1/5 of the book on Wednesday. Andy says that altogether he read more of the book on Tuesday and Wednesday than Mike. Is Andy correct? Explain your thinking.

Answer 1

Andy is incorrect

Step-by-step explanation:

Mike and Andy both read
`(1)/(2)` of the entire book on Tuesday, and on Wednesday, Mike read
`(1)/(3)` of the book while Andy only read
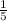`(1)/(5)` of it. Since the fraction
`(1)/(3)` is greater than
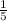`(1)/(5)`, that means Mike read a greater amount of the book than Andy, so he is wrong. If you'd like further proof, Mike read
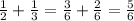`(1)/(2) + (1)/(3) = (3)/(6) + (2)/(6) = (5)/(6)` of the book during Tuesday and Wednesday, while Andy read
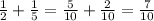`(1)/(2) + (1)/(5) = (5)/(10) + (2)/(10) = (7)/(10)` of the book during Tuesday and Wednesday. To compare the two fractions, you need to make sure they have the same denominator. The least common multiple of 6 and 10 is 30, so that's what the denominator is going to be when we compare the fractions.
`(5)/(6) * (5)/(5) = (25)/(30)`,
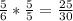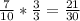`(7)/(10) * (3)/(3) = (21)/(30)`. Since the fractions' denominators are the same, we can just compare the numerators. 25 is greater than 21, so Mike read more of the book on Tuesday and Wednesday than Andy did.