Question

Help pls with answer!!!Rewrite the function in the given form.

Answer 1

`g(x) = (-2)/(x-1)+5\\\\`

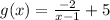
The graph is shown below.

=========================================================

Step-by-step explanation:

Notice that if we multiplied the denominator (x-1) by 5, then we get 5(x-1) = 5x-5.

This is close to 5x-7, except we're off by 2 units.

In other words,

5x-7 = (5x-5)-2

since -7 = -5-2

Based on that, we can then say,

`g(x) = (5x-7)/(x-1)\\\\g(x) = (5x-5-2)/(x-1)\\\\g(x) = ((5x-5)-2)/(x-1)\\\\g(x) = (5(x-1)-2)/(x-1)\\\\g(x) = (5(x-1))/(x-1)+(-2)/(x-1)\\\\g(x) = 5+(-2)/(x-1)\\\\g(x) = (-2)/(x-1)+5`

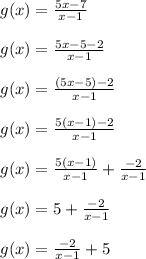
This answer can be reached through alternative methods of polynomial long division or synthetic division (two related yet slightly different methods).

-------------------------

Compare the equation
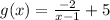`g(x) = (-2)/(x-1)+5\\\\` to the form
`g(x) = (a)/(x-h)+k\\\\`

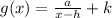
We can see that

• a = -2
• h = 1
• k = 5

The vertical asymptote is x = 1, which is directly from the h = 1 value. If we tried plugging x = 1 into g(x), then we'll get a division by zero error. So this is why the vertical asymptote is located here.

The horizontal asymptote is y = 5, which is directly tied to the k = 5 value. As x gets infinitely large, then y = g(x) slowly approaches y = 5. We never actually arrive to this exact y value. Try plugging in g(x) = 5 and solving for x. You'll find that no solution for x exists.

The point (h,k) is the intersection of the horizontal and vertical asymptote. It's effectively the "center" of the hyperbola, so to speak.

The graph is shown below. Some points of interest on the hyperbola are

• (-1,6)
• (0,7) .... y intercept
• (1.4, 0) .... x intercept
• (2, 3)
• (3, 4)

Another thing to notice is that this function is always increasing. This means as we move from left to right, the function curve goes uphill.