Question

Suppose that the standard deviation of quarterly changes in the prices of a commodity is $0.65, the standard deviation of quarterly changes in a futures price on the commodity is $0.81, and the coefficient of correlation between the two changes is 0.8. A three-month contract is used for hedging. Which of the following is true?

A. The size of the futures position should be 64.2% of the size of the company’s exposure in a three-month hedge.
B. The size of the company’s exposure should be 64.2% of the size of the futures position in a three-month hedge.
C. The size of the futures position should be 35.8% of the size of the company’s exposure in a three-month hedge.
D. The size of the futures position should be 99.7% of the size of the company’s exposure in a three-month hedge.

Answer 1

The size of the futures position should be 64.2% of the size of the company’s exposure in a three-month hedge.

Step-by-step explanation:

As given,

The standard deviation of quarterly changes in the prices of a commodity = $0.65

The standard deviation of quarterly changes in a futures price on the commodity = $0.81

The coefficient of correlation between the two changes = 0.8

Now,

Optimal hedge ratio = 0.8×
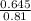`(0.645)/(0.81)` = 0.8×0.80 = 0.6419

⇒Optimal hedge = 0.6419 ≈ 0.642 = 64.2 %

⇒The size of the futures position should be 64.2% of the size of the company’s exposure in a three-month hedge.