Question

Item X is a standard item stocked in a company's inventory of component parts. Each year the firm, on a random basis, uses about 1,600 of item X, which costs $25 each. Storage costs, which include insurance and cost of capital, amount to $6 per unit of average inventory. Every time an order is placed for more of item X, it costs $12. a. Whenever item X is ordered, what should the order size be

Answer 1

80 quantities

Step-by-step explanation:

to get the order size of the item, we have to use this formula

`optimal quantity = \sqrt{(2DS)/(H) }`

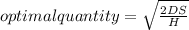
From this formula above,

D = annual demand = 1600

s = set up cost= 12

h = holding cost = 6

`\sqrt{(2*1600*12)/(6) }`

`√(6400) \\=80`

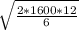
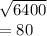
from this solution above, the order size should be 80 quantities

thank you