Question

Assuming the probability of a single sample testing positive is 0.15​, find the probability of a positive result for two samples combined into one mixture. Is the probability low enough so that further testing of the individual samples is rarely​ necessary?

Answer 1

`P(Positive\ Mixture) = 0.2775`

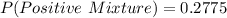
The probability is not low

Explanation:

Given

`P(Single\ Positive) = 0.15`

`n = 2`

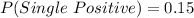
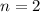
Required

`P(Positive\ Mixture)`

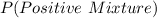
First, we calculate the probability of single negative using the complement rule

`P(Single\ Negative) = 1 - P(Single\ Positive)`

`P(Single\ Negative) = 1 - 0.15`

`P(Single\ Negative) = 0.85`

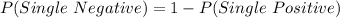
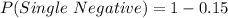
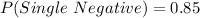
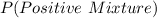`P(Positive\ Mixture)` is calculated using:

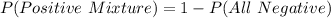`P(Positive\ Mixture) = 1 - P(All\ Negative)` ---- i.e. complement rule

So, we have:

`P(Positive\ Mixture) = 1 - 0.85^2`

`P(Positive\ Mixture) = 1 - 0.7225`

`P(Positive\ Mixture) = 0.2775`

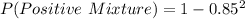
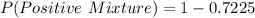
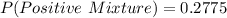
Probabilities less than 0.05 are considered low.

So, we can consider that the probability is not low because 0.2775 > 0.05