Answer:
B) 0.283
Explanation:
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

In which
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

And p is the probability of X happening.
25% of the students drive themselves to school.
This means that
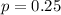
Class of 18 students
This means that

What would be the probability that at least 6 students drive themselves to school?
This is

In which
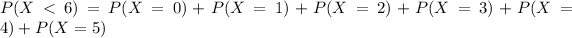
So

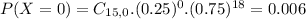

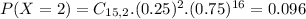
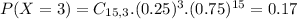
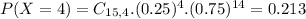
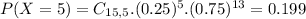
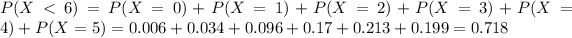
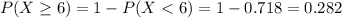
Closest option is B, just a small rounding difference.